Innholdsfortegnelse
Oppgaver
Her er en liste over alle de anbefalte oppgaver og alle prosjektoppgaver.
Anbefalte oppgaver
Limits and Continuity
Section 1.1: Examples of Velocity, Growth Rate, and Area
1.1.5-8, 1.1.12-13.
Section 1.2: Limits of Functions
1.2.1, 1.2.3, 1.2.5, 1.2.9, 1.2.23, 1.2.25, 1.2.55, 1.2.57, 1.2.75 , 1.2.77.
Section 1.3: Limits at Infinity and Infinite Limits
1.3.3, 1.3.13, 1.3.29, 1.3.33.
Section 1.4: Continuity
1.4.1, 1.4.3, 1.4.5 ,1.4.11, 1.4.15, 1.4.17, 1.4.23, 1.4.29, 1.4.31.
Section 1.5: The Formal Definition of Limit
1.5.1, 1.5.5, 1.5.9, 1.5.20.
Differentiation
Section 2.1: Tangent Lines and Their Slopes
2.1.1, 2.1.11, 2.1.16, 2.1.23.
Section 2.2: The Derivative
2.2.3, 2.2.19, 2.2.48, 2.2.55.
Section 2.3: Differentiation Rules
2.3.7, 2.3.29, 2.3.31, 2.3.46.
Section 2.4: The Chain Rule
2.4.5, 2.4.13, 2.4.24, 2.4.27.
Section 2.5: Derivatives of Trigonometric Functions
2.5.16, 2.5.35, 2.5.53, 2.5.58.
Section 2.6: Higher Order Derivatives
2.6.15.
Section 2.7: Using Differentials and Derivatives
2.7.12, 2.7.21, 2.7.23.
Section 2.8: The Mean Value Theorem
2.8.4, 2.8.15, 2.8.21.
Section 2.9: Implicit Differentiation
2.9.5, 2.9.16, 2.9.17.
Section 2.10: Antiderivatives and Initial-Value Problems
2.10.12, 2.10.26, 2.10.42.
Section 2.11: Velocity and Acceleration
2.11.7, 2.11.12.
Løsningsforslag
LF 2.7-2.11
Transcendental Functions
Section 3.1: Inverse Functions
3.1.3, 3.1.7, 3.1.11, 3.1.21, 3.1.25, 3.1.28, 3.1.34.
Section 3.2: Exponential and Logarithmic Functions
3.2.3, 3.2.5, 3.2.15, 3.2.26, 3.2.31.
Section 3.3: The Natural Logarithm and Exponential
3.3.3, 3.3.7, 3.3.15, 3.3.17, 3.3.39, 3.3.45, 3.3.54, 3.3.60.
Section 3.4: Growth and Decay
3.4.1, 3.4.3, 3.4.7, 3.4.11, 3.4.12.
Section 3.5: The Inverse Trigonometric Functions
3.5.11, 3.5.17, 3.5.19, 3.5.29, 3.5.33, 3.5.43.
Section 3.6: Hyperbolic Functions
3.6.2, 3.6.5, 3.6.7, 3.6.9.
More Applications of Differentiation
Section 4.1: Related rates
4.1.1, 4.1.3, 4.1.6, 4.1.18.
Section 4.2: Finding roots of equations
4.2.1, 4.2.3, 4.2.8, 4.2.11.
Section 4.3: Indeterminate forms
4.3.1, 4.3.3, 4.3.12, 4.3.23, 4.3.26, 4.3.32.
Section 4.4: Extreme values
4.4.1, 4.4.3, 4.4.6, 4.4.9, 4.4.19, 4.4.25, 4.4.32.
Section 4.5: Concavity and inflections
4.5.1, 4.5.3, 4.5.4, 4.5.17, 4.5.28, 4.5.35.
Section 4.6: Sketching the graph of a function
4.6.7, 4.6.11, 4.6.16, 4.6.23.
Section 4.7: Graphing with computers
-
Section 4.8: Extreme-value problems
4.8.1, 4.8.2, 4.8.13, 4.8.28, 4.8.31, 4.8.39, 4.8.49.
Section 4.9: Linear approximations
4.9.1, 4.9.3, 4.9.10, 4.9.29.
Section 4.10: Taylor polynomials
4.10.1, 4.10.6, 4.10.9, 4.10.10, 4.10.22, 4.10.27, 4.10.28.
Section 4.11: Roundoff error, truncation error, and computers
-
Integration
Section 5.1: Sums and Sigma Notation
5.1.1, 5.1.3, 5.1.7, 5.1.9, 5.1.11, 5.1.16, 5.1.17, 5.1.21, 5.1.24, 5.1.32.
Section 5.2: Areas as Limits of Sums
5.2.3, 5.2.12, 5.2.19.
Følgende Maple-regneark kan være nyttig når du skal regne ovennevnte oppgaver::Riemannsums
Section 5.3: The Definite Integral
5.3.1, 5.3.2, 5.3.9 (you may want to use the result from exercise 5.1.39 in exercise 5.3.9).
Section 5.4: Properties of the Definite Integral
5.4.1, 5.4.7, 5.4.9, 5.4.23, 5.4.27, 5.4.33, 5.4.42.
Pencast med løsning til oppgave 5.4.42
Section 5.5: The Fundamental Theorem of Calculus
5.5.1, 5.5.6, 5.5.7, 5.5.11, 5.5.14, 5.5.23, 5.5.33, 5.5.39,41, 5.5.44, 5.5.49.
Pencast med løsning til oppgave 5.5.14
Pencast med løsning til oppgave 5.5.44
Section 5.6: The Method of Substitution
5.6.1, 5.6.2, 5.6.5, 5.6.7, 5.6.8, 5.6.12, 5.6.21, 5.6.22, 5.6.45.
Pencast med løsning til oppgave 5.6.12
Pencast med løsning til oppgave 5.6.22
Følgende Maple-regneark kan være nyttig når du skal regne ovennevnte oppgaver: :Substitutions
Section 5.7: Areas of Plane Regions
5.7.1, 5.7.6, 5.7.29.
Pencast med løsning til oppgave 5.7.6 (OBS! Regnefeil i nest siste linje. Svaret skal bli 9 kvadratenheter).
Techniques of Integration
Section 6.1: Integration by Parts
6.1.1, 6.1.4, 6.1.13, 6.1.33, 6.1.37.
Relevant Maple Worksheet: -:Partial integration
Section 6.2: Integrals of Rational Functions
6.2.1, 6.2.3, 6.2.7, 6.2.9, 6.2.20, 6.2.27.
Relevant Maple worksheet: -:Rational Functions
Section 6.3: Inverse Substitutions
6.3.1, 6.3.8, 6.3.43, 6.3.46, 6.3.49, 6.3.51.
Relevant Maple worksheet: -:Substitutions
Section 6.4: Other Methods for Evaluating Integrals
6.4.1, 6.4.3.
Relevant pencast: The method of undetermined coefficients
Section 6.5: Improper Integrals
6.5.1, 6.5.3, 6.5.5, 6.5.6, 6.5.27, 6.5.31.
Section 6.6: The Trapezoid and Midpoint Rules
6.6.1, 6.6.5.
Section 6.7: Simpson's Rule
6.7.1, 6.7.5.
Relevant Maple worksheet: -:Numerical Integration
Section 6.8: Other Aspects of Approximate Integration
6.8.1, 6.8.7, 6.8.9, 6.8.10.
Applications of Integration
Section 7.1: Volumes by Slicing – Solids of Revolution
7.1.1, 7.1.5, 7.1.7, 7.1.11, 7.1.18.
Relevant pencasts:
Relevant Maple worksheet: :Volumes of revolution
Section 7.2: More Volumes by Slicing
7.2.1, 7.2.15.
Relevant pencasts:
Section 7.3: Arc Length and Surface Area
7.3.1, 7.3.7, 7.3.15, 7.3.23, 7.3.24, 7.3.34, 7.3.37.
Relevant pencasts:
Relevant Maple Worksheet: :Arclength
Section 7.4: Mass, Moments, and Centre of Mass
7.4.1, 7.4.2, 7.4.3, 7.4.4, 7.4.16.
Relevant pencasts:
Relevant Maple worksheet: :Centre of Mass
Section 7.5: Centroids
7.5.1,2, 7.5.15, 7.5.23.
Section 7.6: Other Physical Applications
7.6.1, 7.6.3, 7.6.6.
Section 7.7: Applications in Business, Finance and Ecology
7.7.1, 7.7.9, 7.7.17.
Differential Equations
Section 7.9: First-Order Differential Equations
7.9.1, 7.9.6, 7.9.7, 7.9.11, 7.9.13, 7.9.19, 7.9.21, 7.9.28, 7.9.31.
Section 18.1: Classifying Differential Equations
18.1.1, 18.1.3.
Section 18.3: Existence, Uniqueness, and Numerical Methods
18.3.1-3, 18.3.4-6, 18.3.13, 18.3.15, 18.3.16.
Sequences, Series and Power Series
9.1 Sequences and Convergence
9.1.1, 9.1.3, 9.1.24, 9.1.31, 9.1.34, 9.1.36.
9.2 Infinite Series
9.2.1, 9.2.10, 9.2.11, 9.2.16, 9.2.19, 9.2.21.
9.3 Convergence Tests for Positive Series
9.3.1, 9.3.4, 9.3.7, 9.3.9, 9.3.18, 9.3.28, 9.3.38.
-:Convergence tests
-:Problem 9.3.28
9.4 Absolute and Conditional Convergence
9.4.1, 9.4.5, 9.4.13, 9.4.20.
9.5 Power Series
9.5.1, 9.5.5, 9.5.12, 9.5.13, 9.5.15, 9.5.21.
-:Power series
-:Problem 9.5.12
9.6 Taylor and Maclaurin Series
9.6.1, 9.6.2, 9.6.7, 9.6.29, 9.6.37.
9.7 Applications of Taylor and Maclaurin Series
9.7.1, 9.7.15, 9.7.21, 9.7.24.
Prosjektoppgaver
Semesteruke 1 (26.-30. aug)
These problems are to be presented 26.-30. August. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1
Define the function \(f\) by
\[f(x) = \begin{cases} 1 & \text{for} \,\, x \,\, \text{rational} \\ 0 & \text{for} \,\, x \,\, \text{irrational.} \end{cases} \]
At which points is \(f\) continuous/discontinuous?
Hint 1: You will have to use the \(\varepsilon\)-\(\delta\) definition of continuity.
Hint 2: Use the following property of the real numbers: For any two rational numbers \(x<y\), there exists an irrational number \(p\) such that \(x<p<y\). Similarly, for any two irrational numbers \(x<y\), there exists a rational number \(r\) such that \(x<r<y\). (Remark: From this property, one might think that there are an equal amount of rational and irrational number, but, in a precise sense, the set of irrational numbers is much, much larger than the set of rational numbers!)
Problem 2
Suppose \(f\) is a continuous function on the interval \([0,1]\), and \(f(0)=f(1)\).
a) Show that \(f(a)=f(a+1/2)\) for some \(a\in [0,1/2]\).
Hint: Let \(g(x)=f(x+1/2)-f(x)\), and use the Intermediate-Value Theorem.
b) If \(n\) is an integer larger than 2, show that \(f(a)=f(a+1/n)\) for some \(a\in [0,1-1/n]\),
Problem 3
Consider the functions \(f\) and \(g\) defined by \[f(x)=\frac{a\cos(x)+bx}{cx}\] for real numbers \(a,b,c\) so that \(c \neq 0\) and \[g(x)=\sqrt{(ax)^2+bx+c\sin(x)}-ax\] for real numbers \(a,b,c\) so that \(a>0\)
By using Maple, make plots of the functions \(f\) and \(g\) for different values of \(a,b,c\) and try to guess what the limits are when \(x\to\infty\). Also try to compute the limits in Maple.
Finally, calculate the limits by hand (without using L'Hosptal's rule). Hint: Use the Squeeze Theorem.
Problem 4
Let \(f\) be a continuous function, with domain the real numbers.
a) Given a closed interval \([a,b]\). Show that the set \(A = f([a,b]) = \{f(x) \, | \, x \in [a,b]\}\), i.e. the set of all numbers \(f(x)\) where \(x\) ranges over \([a,b]\), is also a closed interval.
b) Let \((a,b)\) be an open interval. Define the set of real numbers \(B = \{ x \, | \, f(x) \in (a,b) \}\), i.e. the set of all points \(x\) such that \(f(x)\) is a number in \((a,b)\). Suppose \(c\) is in \(B\). Show that there is an open interval \((c-h, c+h)\) centered in \(c\) which is a subset of \(B\), i.e. all numbers in \((c-h,c+h)\) are also in \(B\). (Sets like \(B\), such that if it includes some point, it also contains an open interval centered in that point, are called open sets.)
Hint: use the \(\varepsilon\)-\(\delta\) definition of continuity of \(f\) at the point \(c\).
c) Find a discontinuous function which doesn't satisfy the property in (a). Same for (b).
Semesteruke 2 (2.-6. sep)
1. Oscillations and derivatives
Define the function \(g\) by \(g(x) = x^2\sin\frac 1x\) for \(x\neq 0\) and \(g(0) = 0\). Is \(g\) continuous in \(x=0\)? Differentiable in \(x=0\)? Continuously differentiable in \(x=0\)?
2. Using Maple to find derivatives
(Exercise 61 for chapter 2.5 in Adams.)
Let \(f(x) = \sqrt{2x^2+3\sin(x^2)} -\frac{(2x^2+3)^{3/2}\cos(x^2)}{x}\).
Use Maple to find \(f'(\sqrt \pi)\). Also use Maple to find the tangent at \(x= \sqrt \pi \).
3. Logarithmic derivation
In this exercise we will derive a useful method for calculating the differentials of complex expressions, called logarithmic differentiation.
a) Use the chain rule to show that if \(f\) is differentiable and \(f(x) \neq 0\), then
\(f'(x) = f(x)[\ln|f(x)|]' \).
b) Let \(f\) and \(g\) be two differentiable functions, and assume that \(f\) is positive. Show that \([f(x)^{g(x)}]' =f(x)^{g(x)}\left[g'(x)\ln f(x) +\frac{g(x)f'(x)}{f(x)}\right] \)
4. Tangents to polynomials
Let \(P(x)\) be a polynomial and let the straight line \(y=l(x)\) be the tangent to \(P(x)\) at \(x=0\). Show that \(P(x)-l(x)\) is a polynomial with no constant term and no first degree term.
Semesteruke 3 (9.-13. sep)
1. Lipschitz continuous functions
A function \(f\) is said to be Lipschitz continuous if there exists an \(L>0\) such that for all \(x\) and \(y\) we have \(|f(x)-f(y)|\leq L|x-y|\). The constant \(L\) is called a Lipschitz constant of \(f\).
a) Give an example of a Lipschitz continuous function.
b) Show that Lipschitz continuity implies continuity.
c) Show that if \(f\) is a differentiable function with bounded derivative (that is, there exists an \(L\) such that \(|f'(x)|\le L\) for all \(x\)), then \(f\) is Lipschitz continuous. (Hint: The Mean-Value Theorem)
2. Counterexamples
a) Find a continuous function \(f\) on \([-1,1]\) such that \(f\) has a maximal value at \(c\in (-1,1)\), but \(f'(c)\) does not exist.
b) Find a continuous function \(f\) on \([-1,1]\) such that \(\frac{f(1)-f(-1)}{1-(-1)} \neq f'(x)\) for any \(x\in(-1,1)\) where \(f'(x)\) is defined.
3. Driving a car
A car is driving at night along a level, curved road. It starts in the origin, the equation of the road is \(y = x^2 \), and the car's x-coordinate is an increasing function of time. There is a signpost located at \((2,3.75)\).
a) What is the position of the car when its headlight illuminates the signpost? Do you have any implicit physical assumptions in your solution?
b) What is the shortest distance between the signpost and the car? (You will need to solve a third degree equation in order to answer this question. Either use Maple to do that or explain what you would do if you had a solution to the third degree equation).
c) Let \( \frac{\mathrm{d}x}{\mathrm{d}t}= v_x\) and \(\frac{\mathrm{d}y}{\mathrm{d}t}=v_y.\) The car's velocity is then \([v_x, v_y]\). How are \(v_x\) and \(v_y\) related?
4. Ellipse and Parabola
Show that the ellipse \(\frac{1}{3}x^2 + y^2=1 \) and the hyperbola \(x^2 - y^2 =1 \) intersect at right angles. Use Maple to illustrate the situation.
Semesteruke 4 (16.-20. sep)
These problems are to be presented 16-20 September. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1
a) Let \(f(x) =\mathrm{e}^x\) with domain \((-\infty,\infty)\), and \(g(x)=x^2\) with domain \([0,\infty)\). Show that \((f\circ g)^{-1}(x) =\sqrt{\ln x}\), and show that \(g^{-1}(f^{-1}(x)) =\sqrt{\ln x}\).
b) In general, let \(f\) and \(g\) be functions with inverses \(f^{-1}\) and \(g^{-1}\) respectively. Show that \((f\circ g)^{-1} = g^{-1}\circ f^{-1}\).
Hint: Solution to Exercise 3.1.34.
Problem 2 (Problem 75, p.183 in Adams)
We will show that \(2<\mathrm{e} <3\). Let \(f(t) = 1/t \) for \(t>0\).
a) Show that the area bounded by \(y =f(t)\), \(y =0\) and \(t=1\), \(t=2\) is less than \(1\). Deduce from this that \(\mathrm{e} >2\).
b) Show that all tangent lines to the graph of \(f\) lies below the graph. (Hint: Show that \(f''(t) >0\) for all \(t\).)
c) Find the lines \(T_2\) and \(T_3\) that are tangent to \(y =f(t)\) at \(t=2\) and \(t=3\) respectively.
d) Find the area \(A_2\) enclosed by \(y=T_2\) , \(y=0\) and \(t=1\), \(t=2\). Also find the area \(A_3\) enclosed by \(y=T_3\) , \(y=0\) and \(t=2\), \(t=3\).
e) Show that \(A_2+A_3 >1\), and deduce that \(\mathrm{e}<3\).
Problem 3
The number of people infected by a virus at time \(t\) is given by \[ y(t) =\frac{L}{1+M\mathrm{e}^{-kt}}\] for \(t \geq 0\), where \(t\) is measured in months.
a) Assuming that we at the start of the outbreak had 200 patients, and after 1 month there were 1000 people infected. Eventually, the number of patients stabilizes at 10 000. Use this information to find the constants \(L, M\) and \(k\).
b) How many people were infected after 3 months? How fast was he infection spreading at this time?
c) At what point was the number of patients growing fastest?
Semesteruke 5 (23.-27. sep)
These problems are to be presented 23-27 September. See here for where you should meet, and here to sign up for presenting a problem.
PDF-version of the project problems
Problem 1
Let the function \(f\) be defined by \[f(x) = \begin{cases} x\tanh(x) \ \text{for} \ x \geq 0 \\ ax^2+bx+c\ \text{for} \ x <0. \end{cases} \]
Find the constants \(a, b, c\) so that \(f\) is two times differentiable.
Problem 2
A water tank shaped like a cone pointing downwards is 10 metres high. 2 metres above the tip the radius is 1 metre. Water is pouring from the tank into a cylindrical barrel with vertical axis and a diameter of 8 metres. Assume that the height of the water in the tank is 4 metres, and is decreasing at a rate of 0.2 metres per second. How fast is the height of the water in the barrel changing?
Problem 3
The fuel consumption of a car, \(D\) measured in litres per hour, is related to the velocity \(v\) by the following formula\[D(v) = \frac{\mathrm{e}^v+\mathrm{e}^{-v}}{2}+\frac{1}{v},\] where \(v\) is measured in kilometres per hour.
a) Find an equation describing the velocity \(v_0\) that gives minimal fuel consumption.
b) Use Newton's Method to give an approximated solution the the equation found in a).
Problem 4
a) Prove that the polynomial \(p(x) = x^5+7x^3-20\) has exactly one root in the interval \((1, 2\)).
Hint: See the example Newton's Method.
b) Write a script in Maple to implement Newtons Method to find an approximated value for this root. For this and problem c) it may be useful to take a look at : Newton's Method.
c) Use Maples Student[Calculus]-package to approximate this root. How does the value compare to the one found in b)?
Semesteruke 6 (30. sep - 4. okt.)
These problems are to be presented 30 September-4 October. See here for where you should meet, and here to sign up for presenting a problem.
PDF-version of the project problems}
Problem 1 (Problem 4.9.48 in Adams)
A sector is cut out of a disk of radius \(R\), as shown below. The remaining part of the disk is bent up so that the two edges join and a cone is formed. What is the largest possible volume for the cone?

Problem 2
The limit \[\lim_{x \to 0}\left(\frac{1}{\sin(px)}-\frac{1}{\mathrm{e}^{x/2}-1}\right)\] exists for exactly one real-valued \(p\). Find this value, and calculate the limit.
Problem 3
Adams defines a concave up, or convex, function on an interval \(I\) to be a differentiable function such that the derivative \(f'\) is an increasing function on \(I\). In this problem, we will show that for differentiable functions this is equivalent to the more general definition of a convex function. We will prove the following:
Assume \(f\) is differentiable on an interval \(I\). Then \(f'\) is an increasing function on \(I\) if and only if for all \(x, y \in I\) and all \(t \in [0,1]\) we have that \[f((1-t)x+ty)\leq (1-t)f(x)+tf(y).\]
a) First make the substitution \(x_1=x\), \(x_3 =y\) and \(x_2 =(1-t)x_1 +tx_3\) to prove that if the inequality above holds, then for any \(x_1, x_2, x_3\) in \(I\) such that \(x_1 < x_2 <x_3\), we have \[\frac{f(x_2)-f(x_1)}{x_2-x_1}\leq \frac{f(x_3)-f(x_2)}{x_3-x_2}.\] HINT: You will need to solve for \(t\) in the substitution.
b) Now assume that \[\frac{f(x_2)-f(x_1)}{x_2-x_1}\leq \frac{f(x_3)-f(x_2)}{x_3-x_2}.\] holds for \(x_1 < x_2 <x_3\). Show that this implies that the inequality \[f((1-t)x+ty)\leq (1-t)f(x)+tf(y).\] holds for \(x, y \in I\) and all \(t \in [0,1]\).
c) Now suppose \(f'\) increases on \(I\). Use the result from a) and b), and the Mean Value theorem to conclude that \[\frac{f(x_2)-f(x_1)}{x_2-x_1}\leq \frac{f(x_3)-f(x_2)}{x_3-x_2},\] and equivalently, \[f((1-t)x+ty)\leq (1-t)f(x)+tf(y).\]
d) For the other implication, suppose that for \(x_1 < x_2 <x_3\), we have \[\frac{f(x_2)-f(x_1)}{x_2-x_1}\leq \frac{f(x_3)-f(x_2)}{x_3-x_2}.\] Prove that \(f'\) is increasing on \(I\), and that the theorem holds.
Problem 4
a) Let \(f(x)= x^{10}\). Use Maple or other software to plot \(f(x)-\sqrt{f(x)^2}\) on the intervals \((-10, 10)\) and \((-\infty, \infty)\). Why does the plot indicate that the function is not identically equal to 0?
b) Assume that \(x>0\), and use Maple to calculate different values of \(f(x)-\sqrt{f(x)^2}\). Also use Maple to simplify the expression. Why does this answer differ from the one indicated in a)?
c) Use the function \(g(x)=2^x\ln(1+2^{-x})\) to determine the machine epsilon for your computer. What does the answer tell you? How does this relate to point a)?
Semesteruke 7 (7.-11. okt.)
These problems are to be presented during October 7-11. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1
a) Calculate the \(n\)'th order Taylor polynomial for \(\mathrm{e}^x\) at \(x=0\).
b) Let \(s_n =\sum_{i=0}^{n}\frac{1}{i!}.\) Use the polynomial found in a) and Lagrange's remainder to show that \[\mathrm{e}= \lim_{n \to \infty}s_n=\sum_{i=0}^{\infty}\frac{1}{i!}.\]
Problem 2
We want to show that the volume of a cone with height \(h\) and radius \(r\) is \(\pi r^2h/3 \), by approximating the volume with \(n\) cylindrical boxes with height \(h/n\), shaded green in the figure below.
a) Show that the volume of the green figure depicted in Figure 1 is \[V_{out}=\pi\left(\frac{r}{n}\right)^2\left(\frac{h}{n}\right)+\pi\left(\frac{2r}{n}\right)^2\left(\frac{h}{n}\right)+\cdots +\pi r^2\left(\frac{h}{n}\right).\]
b) Show that the volume of the green figure depicted in Figure 2 is \[V_{in}= 0+\pi\left(\frac{r}{n}\right)^2\left(\frac{h}{n}\right)+\pi\left(\frac{2r}{n}\right)^2\left(\frac{h}{n}\right)+\cdots +\pi \left(\frac{(n-1)r}{n}\right)^2\left(\frac{h}{n}\right).\]
c) Use a) and b) to prove that \(V=\pi r^2h/3\). You may find it useful that \(\sum\limits_{i=0}^{n}i^2 =n(n+1)(2n+1)/6\).
Problem 3 (Exam 1996 in 75001, problem 6)
Let \[f(x)=\frac{1}{\sqrt{1-x}}\] for \(x<1\).
a) Use induction (see page 110 in the textbook) to show that \[f^{(n)}(x)=\frac{(2n)!}{2^{2n}n!(1-x)^{(2n+1)/2}}\] for \(n=1,2,3,\dots\) (recall that \(n!=n(n-1)(n-2)\cdots 1\) and \((2n)!=2n(2n-1)(2n-2)\cdots 1\), see page 128 in the textbook).
b) Let \(P_n\) be the \(n\)th-order Taylor polynomial for \(f\) about \(a=0\) and let \(R_n(x)=f(x)-P_n(x)\). Find \(P_3(x)\) and \(R_3(x)\) and show that \[|f(x)-P_3(x)|=|R_3(x)|<5\cdot 10^{-5}\] when \(|x|<0.1\).
c) How big does \(n\) have to be in order for \[|f(x)-P_n(x)|=|R_n(x)|<5\cdot 10^{-7}\] when \(|x|<0.1\)?
Problem 4 (Exam 2000 in SIF5003, problem 9)
The equation \[x^2+y\mathrm{e}^y =1\] and the inequality \(y>-1\) uniquely defines a function \(y=f(x)\). Find \(f'(1)\), and calculate the second order Taylor polynomial for \(f\) around \(x=1\).
Semesteruke 8 (14.-18. okt.)
These problems are to be presented during October 14-18. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1
a) Use the substitution \(u=\sqrt[3]{x}\) to show that \[\int\frac{\sqrt[3]{x}}{\sqrt[3]{x}+1}\ dx=\int\frac{3u^3}{u+1}\ du\Big\rvert_{u=\sqrt[3]{x}}.\]
b) Use polynomial division to show that \[\frac{u^3}{u+1}=u^2-u+1-\frac{1}{u+1}.\]
c) Calculate the integral \[\int\frac{\sqrt[3]{x}}{\sqrt[3]{x}+1}\ dx.\]
Problem 2 (2: Exam 2011 in TMA4100, problem 6)
A boat lies at distance \(a\) from the wharf, and is moored with a rope at the point \(O\). A girl takes the rope, and walks along the wharf while dragging the boat by the rope, which we assume is taunt all the time. The prow of the boat follows the dotted line as shown in the figure.
We want to describe the dotted line as a graph \(y=f(x)\), for \(0< x \leq a\).
a) Show that \(y=f(x)\) satisfies \[y'=-\frac{\sqrt{a^2-x^2}}{x}.\]
b) Solve this equation by integrating it, and find the function \(f\). Use the substitution \(u=\sqrt{a^2-x^2}.\) You may also use that \[\int \frac{1}{a^2-u^2} \ du=\frac{1}{a}\ln\left(\frac{a+u}{\sqrt{a^2-u^2}}\right)+C \] for \(|u|<a.\)
Problem 3
a) Express the area of the unit disk, i.e. the area enclosed by the unit circle \(x^2+y^2 = 1\), as a definite integral.
b) Compute the definite integral in (a) using the substitution \(x = \cos(\theta)\). Is the value of the integral reasonable?
c) Use integration to find the area enclosed by the ellipse \(\frac{x^2}{9}+ \frac{y^2}{16} = 1\). (Hint: substitution, and the integral in (a).)
Problem 4 ( Exam 1993 in 75011, problem 4)
a) Find a function \(f\) so that \[\sum_{i=1}^{n}\frac{1}{n\left(2+\frac{i}{n}\right)\ln\left(2+\frac{i}{n}\right)}\] is a Riemannsum for \(f\) on the interval \([0,1]\).
b) Calculate the limit \[\lim_{n \to \infty}\sum_{i=1}^{n}\frac{1}{n\left(2+\frac{i}{n}\right)\ln\left(2+\frac{i}{n}\right)}\].
Semesteruke 9 (21.-25. okt.)
These problems are to be presented during October 21-25. See here for where you should meet, and here to sign up for presenting a problem.
PDF-version of the exercises
Problem 1 (Maple TA)
Find the smallest positive number \(x\) such that \(F(x) = \frac{1}{20 \pi}\) where \(F\) is given by \[F(x) = \int_0^x e^{5\sqrt{3}\pi t}\sin(15\pi t)\,dt.\]
Your answer should be an exact positive rational number.
Hint: 1) Try first to find an antiderivative, \(G(t)\), of the integrand by using integration by parts twice.
2) Then \(F(x) = G(x) - G(0).\)
3) You may find it useful to remember that \(\tan\pi/3 = \sqrt{3}.\)
Problem 2 (Exam 1996 in 75011, problem 2)
a) Calculate the indefinite integral \[\int \frac{2}{(x+1)(x^2+1)} \ dx.\] Hint: :Rational Functions and Problem 6.2.20.
b) Find the exact value of the improper integral \[\int\limits_{1}^{\infty} \frac{2}{(x+1)(x^2+1)} \ dx.\]
Hint: Another example of an improper integral
Problem 3 (Exam in MAT1001, UiO)
Find \(a>0\) so that the integral \[\int\limits_{0}^{\infty}\cos x \mathrm{e}^{-ax} \ dx \] has maximal value. Find this value. Hint: :.Partial integration, and example 4 page 335 in Adams.
Problem 4 (Coulomb's Law)
Two electrically charged particles repel each other if they have the same charge, and attract each other if they have opposite charge. According to Coulomb's law the force of attraction/repulsion is given by \[F=k\frac{q_1q_2}{r^2}\] where \(k\) is a constant, \(q_1\) and \(q_2\) is the charge of the particles and \(r\) is the distance between them. The work required to move one particle from a distance \(a\) to a distance \(b\) away from the other is given by \[W=-\int\limits_{a}^{b}F(r) \ dr.\]
a) Assume that the particles are at a distance \(d\) from each other, and have opposite charge. How much work is required to move one particle infinitely far from the other?
b) Assume again that the particles are at a distance \(d\) from each other, and have the same charge. How much work is required to move the particles together?
Semesteruke 10 (28. okt. - 1. nov.)
These problems are to be presented October 28 to November 1. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1
The triangular region with vertices \((0, -1)\), \((1, 0)\) and \((0, 1)\) is rotated about the line \(x=2\). Find the volume of the region so generated.
Problem 2: Exam 1999 in SIF 5003, problem 3
a) Let \(f(x)=\sqrt{1+x^4}.\) Find the maximal and minimal value of \[f''(x)= \frac{2x^2(x^4+3)}{(x^4+1)^{3/2}}.\]
b) Use the Trapezoid method with 4 subintervals to find an approximation, \(T_4\), to \[I=\int_{0}^{2} \sqrt{1+x^4} \, dx.\] Use problem a) to find the smallest number \(\epsilon\) so that you can guarantee that the error, \(|T_4-I|\), in the Trapezoid approximation used above is less that \(\epsilon\). Hint: -:Numerical Integration.
Explain why the Trapezoid method always gives a bigger value for the above integral, no matter how many subintervals we choose.
Problem 3: Drilling a hole in a sphere
A sphere has radius 5 cm. We drill a hole with radius 3cm through the center of the sphere. We want to calculate the volume of the solid that remains.
a) Show that the volume of the solid that remains can be written as
\[V=4\pi\int_{3}^{5}x\sqrt{5^2-x^2} \, dx.\] Hint: Draw the situation, and :Volumes of revolution.
b) Calculate the volume of the solid.
Problem 4: Exam 1999 in SIF 5003, problem 9
A water tank is made by rotating the graph of the function \(x=g(y)\) about the \(y\)-axis. The volume of the tank at height \(y\) is then given by \[V(y) =\pi\int_{0}^{y}g(u)^2 \, du.\] A hole is made in the bottom of the tank. According to Torricelli's Law the volume then satisfies \[\frac{\mathrm{d}V}{\mathrm{d}t} =-k\sqrt{y},\] where \(y\) is the height of the water and \(k\) is some positive constant.
Determine the function \(g(y)\) when it is given that the change of the height of water per unit time is constant (that is, \(\frac{\mathrm{d}y}{\mathrm{d}t}\) is constant.) and the volume \(V\) is 1 when \(y=1\).
Semesteruke 11 (4.-8. nov.)
These problems are to be presented November 4-8. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1: Maple TA
The semicircular plate \(x^2+y^2 \leq a^2\), \(y \geq0\) has mass \(m=4\pi\) and center of mass in \( (0, \frac{3}{\pi}) \). If the density at a distance \(s\) from the origin is given by \( \rho(s) =ks\), find \(k\) and the radius \(a\).
Hint: Example 2, page 418 in Adams, and :Centre of Mass.
Problem 2:Exam 1997 in SIF 5003, problem 4
Define the function \(F\) by \[F(x) =\int_{1}^{x}\sqrt{t^3-1}\, dt, \] for \(x \geq 1\).
a) Find the length of the curve \(y =F(x)\) when \(1\leq x\leq 2\). Hint: :Arclength.
b) Find the area of the object we get when we revolve the curve from a) about the line \(x=1\).
Problem 3: Swimming Pool
a) A pool 20 m long and 8 m wide has a sloping plane bottom so that the pool is 1 m deep at one end and 3 m deep at the other end. Find the total force exerted on the bottom if the pool is full of water.
b) Find the total work that must be done to pump all the water out of the pool.
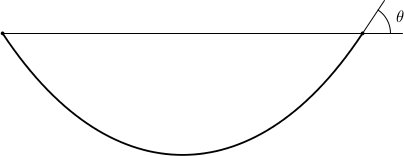
Problem 4: Exam 2002 in SIF 5003, problem 5
a) Let \(a\) be a positive constant. Find the length of the curve \[y=\frac{\cosh{ax}}{a} \] for \(-1\leq x\leq 1\).
b) Explain why the equation \(x\tanh{x}=1\) has exactly one positive solution \(x_0\). Use Newton's Method to find an approximation to \(x_0\).
c)
The curve in problem a) describes a cable of uniform thickness and density which is hanging freely between two points, as illustrated below.
The tensile force (strekkraften) on the cable in the point \(x=1\) equals half the weight of the cable divided by \(\sin{\theta} \), where \(\theta \) is the angle marked on the figure.
What value of \(a\) gives the least tensile force in the point \(x=1\)? How far down does the midpoint of the cable hang, compared to the end points?
Semesteruke 12 (11.-15. nov.)
These problems are to be presented during November 11-15. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1: Maple TA
Given the initial value problem
\[y' +\frac{y}{\tanh{x}} = \ln{2}\cdot\cosh(x) , \ y(1)=b, \]
find the value of b that ensures that y(0) exists.
HINT: Integrating factor, Method 1 page 450 in Adams, and -:Ordinary differential equations.
Problem 2: Exam 1997 in SIF 5003, problem 5
The freezing point \(T\) for water with ionic concentration \(x\), satisfies the differential equation
\[\frac{dT}{dx} =\frac{-aT^2}{1+bx}, \ T(0)=T_0, \]
where \(a\), \(b\) and \(T_0\) are constants with values \(a = 2.49\cdot 10^{-5} \, \mathrm{K}^{-1} \mathrm{M}^{-1}\), \(b= 0.018 \, \mathrm{M}^{-1}\) and \(T_0 = 273.15 \, \mathrm{K}\), where \(\mathrm{M}=\) mol and \(\mathrm{K}=\) Kelvin.
a) Use the differential equation to find the tangent to \(T(x)\) in the point \((0, T_0)\). Use this tangent to find an approximate value to \(T(1.2)\).
b) Solve the initial value problem. Use the solution to find \(T(1.2)\), and compare this value with the value you found in a).
HINT 1: -:Ordinary differential equations.
HINT 2: Differential equations videos.
Problem 3: Continuation Exam 2006 in TMA4100, problem 5
Consider the initial value problem
\[\frac{dy}{dx} = x+y^2,\] with \(y(0)=1\).
a) Use Euler's Method with step-length \(h=0.1\) to find an approximation to \(y(0.3).\)
HINT 1: :Numerical methods.
HINT 2: Differential equations videos.
b) Let \(P_2(x)\) denote the second order Taylor polynomial for the solution of the initial value problem \(y(x)\) at \(x=0\). Find \(P_2(0.3)\). HINT: Differentiate the differential equation implicitly to find \(y''\).
Problem 4: Modeling fish population
A lake has a population of \(x(t)\) fish at time \(t\). Assume that the probability of one fish meeting another in a small time interval is proportional with the population size.
a) Assume that the rate of birth in the population is proportional with the number of random encounters between two fish, and that the rate of death is proportional with the population size. Show that these assumptions leads to the equation
\[\frac{dx}{dt}=bx^2-ax,\] where \(a, b\) are positive constants. Give an interpretation on the terms \(bx^2\) and \(ax\).
b) Assume that the initial population is \(x(0)=x_0\), and find \(x(t)\).
c) Show that there exists a constant \(k_0\) so that if \(x_0 <k_0\), then the population will eventually die out, while if \(x_0>k_0\) the population will grow infinitely large in finite time. Find \(k_0\) and this finite time. What happens if \(k_0=x_0\)?
Semesteruke 13 (18.-22. nov.)
These problems are to be presented November 18-22. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1: Maple TA
Consider the sum
\[\sum_{n=1}^{\infty} \frac{1}{2n-1}\left(\frac{ax+3}{-4}\right)^n,\] where \(a\) is a positive, real number.
Determine the values of \(x\) for which the series
i) converges absolutely,
ii) converges conditionally,
iii) diverges.
Hint: :Convergence tests
Problem 2: Exam 1998 in SIF 5003, problem 6
a) Show that the series \[\sum_{n=2}^{\infty} (-1)^{n+1}\left(\frac{n-1}{n^2}\right)\] converges. Is the convergence absolute or conditional?
b) The partial sum \[S_9 = \sum_{n=2}^{9} (-1)^{n+1}\left(\frac{n-1}{n^2}\right)\]
is an approximation to the sum \(S\) of the series in a). What can you say about \(|S_9-S|\)?
Problem 3: Induction
Use induction to show that \[\sum_{i=0}^{n} x^i =\frac{1-x^{n+1}}{1-x}\] for \(n\geq 1\) and \(x \neq 1\).
Hint: Pink box, page 110 in Adams.
Problem 4: Exam 2003 in TMA4100, problem 10
A figure is made in the following way: We start with a rectangle with sides \(x\) and \(y\), and then add a smaller rectangle with sides \(\frac{1}{2}x\) and \(\frac{1}{2}y\), and then add another with sides \(\frac{1}{4}x\) and \(\frac{1}{4}y\) and so forth, ad infinitum, as indicated in the figure below.
The circumference of the figure is 6. What values of \(x\) and \(y\) makes the area maximal?
Hint: :Power series
Semesteruke 14 (25.-29. nov.)
These problems are to be presented November 25-29. See here for where you should meet, and here to sign up for presenting a problem.
Problem 1: Maple TA
Find the sum of the series
\[\sum_{n=2}^{\infty} \frac{3}{n(n-1)7^n}\]
HINT: First find the sum of
\[\sum_{n=2}^{\infty} \frac{3x^n}{n(n-1)}\] by differentiating the series twice, and then integrating again. See also :Problem 9.5.12.
2: Exam December 2005, problem 7
a) Find the Taylor series at \(x=0\) for the function
\[\frac{\cos{x}-1}{x^2}. \] For which \(x\) does the series converge? You may use that \[\cos(x)=1-\frac{x^2}{2!}+\frac{x^4}{4!}+\cdots +(-1)^{n}\frac{x^{2n}}{(2n)!} \cdots\]
b) Use the series you found to calculate the integral \[\int_{0}^{1}\frac{\cos{x}-1}{x^2} \, dx\] with an absolute error less than \(10^{-4}\). Hint: :Problem 9.3.28.
3: Exam December 2009, problem 3
For which \(x\) does the series \[\sum_{n=1}^{\infty}\frac{(x+1)^n}{n2^n}\] converge?
4: Exam August 2013, problem 5
Consider the series
\[\sum_{n=1}^{\infty}\frac{x^{n+1}}{n} \]
a) Determine the radius of convergence \(R\) for the series, and determine if the series converges for \(\pm R \).
Hint: :Convergence tests.
b) Let \[f(x) = \sum_{n=1}^{\infty}\frac{x^{n+1}}{n}, \] for \(-R<x<R\). Find a closed expression for \(f\). HINT: Write \(f(x)=xg(x)\), and find a closed expression for \(g'(x)\).
5: Exam December 2011, problem 7
a) Integrate the geometric series
\[\frac{1}{1-x^2}=\sum_{n=0}^{\infty} x^{2n}, \, \, |x|<1 \] to show that
\[\frac{1}{2}\ln\left(\frac{1+x}{1-x}\right)=\sum_{n=0}^{\infty}\frac{x^{2n+1}}{2n+1}.\]
b) Show that for \(0<x<1\) we have that
\[\left|\frac{1}{2}\ln\left(\frac{1+x}{1-x}\right)-\sum_{n=0}^{N}\frac{x^{2n+1}}{2n+1}\right|<\frac{x^{2N+3}}{2N+3}\left(\frac{1}{1-x^2}\right), \] and use this to calculate \(\ln{2} \) with an error less than \(10^{-5}\).
6: Exam December 2010, problem 7
Show that the series \[\sum_{n=0}^{\infty}\frac{x^{3n+2}}{(3n+2)n!} \] converges for all \(x\) and that the sum is \[\int_{0}^{x}t\mathrm{e}^{t^3} \, dt. \]
7: Exam August 2010, problem 6
For what values of \(x\) does the series \[\sum_{n=0}^{\infty} \left(\frac{nx}{1+n}\right)^n \] converge?