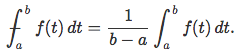TMA4305 Partielle differensialligninger 2021

I do, from time to time, employ terminology and notation that deviates from the book. This page is an attempt to list these cases. Many of them (but not all) are more or less standard in the literature. \( \def\RR{\mathbb{R}} \def\Cc{C_{\text{c}}} \)

• I sometimes don't use fat symbols (in print) or vector arrows above (in handwriting) for points in \(\RR^n\). But I do it some of the time.
• The open ball centred at \(x_0\in\RR^n\) and radius \(R\) is written \(B(x_0;R)\) in the book. I often write \(B_R(x_0)\) instead. Similarly, I write \(\bar B_R(x_0)\) for the closed ball.
• A neighbourhood (often abbreviated “nbhd”) of a point \(x\in\RR^n\), to me, is any subset of \(\RR^n\) which contains an open ball centred at \(x\). (In the book, only such open balls qualify as neighbourhoods. The way the concept is used, it usually makes little or no difference which one is used. One advantage to my way: An open set is a set which is a neighbourhood of each of its members.)
• Functions of compact support: I write (for example) \(\Cc^\infty(\Omega)\) where the book uses \(C_{\text{cpt}}^\infty(\Omega)\). Beware that many authors use \(C_0^\infty(\Omega)\) here. I dislike this because \(C_0(\Omega)\) usually denotes the space of continuous functions which have the limit \(0\) when you approach the boundary of \(\Omega\) or infinity.
• Direction of axes: Even though I follow the book's convention of putting the \(t\) variable first, as in \((t,x)\), I depart from the book in drawing the \(t\) axis vertically and the \(x\) axis horisontally. For a line or curve in \((t,x)\) space I talk about its speed \(\dot x=dx/dt\) rather than its slope (higher speed means smaller slope with my choice of axes).

I write \[ \frac{\partial u}{\partial t} = u_t = \partial_t u,\quad \frac{\partial^3 u}{\partial t\,\partial x^2} = u_{xxt} = \partial_t\partial_x^2 u \] In particular, \(\partial_t\) is the first order operator more commonly written \(\partial/\partial t\). Note the different order of the variables in the third order example above: \(u_{xxt}\) is the \(t\)-derivative of \(u_{xx}\), which could be written \((u_{xx})_t\), so the order \(u_{xxt}\) is natural. But operators compose from right to left, so we write \(\partial_t\partial_x^2\) for the composed operator. In practice, all our functions will be sufficiently smooth so that the order of partial derivatives does not matter, so we can ignore these distinctions most of the time.

Caution: Subscripts do not always indicate partial derivatives. It should be clear from context when they do not. When \(u_s\) is such a quantity where the subscript is just a subscript, the notation \(\partial_t u_s\) is preferable for the partial derivative of \(u_s\) wrt \(t\). (But I might occasionally be tempted to write \(u_{s,t}\), with a comma separating the “mere subscripts” from the partial derivatives.

Whenever \(P\) is some condition that might be true or false, we define \[[\,P\,]=\begin{cases}1&\text{if \(P\) is true},\\0&\text{if \(P\) is false.}\end{cases}\] I like to stretch this definition a bit and say that \(a[\,P\,]=0\) if \([\,P\,]=0\), even if the expression \(a\) is undefined. Thus \(x^{-1}[\,x\ge1\,]\) is defined for all \(x\in\mathbb{R}\).

For an exposition of the many advantages of the Iverson Bracket, see Donald E. Knuth: Two Notes on Notation, American Mathematical Monthly 99:5 403–422 (1992). (You need to be on the NTNU network to access it.)

I like to extend the notation even further: Traditionally, the indicator function, also known as the characteristic function (though the latter clashes with usages in probability theory), of a set \(S\) is denoted \(\chi_S\). Its definition using the Iverson bracket is then \(\chi_S(x)=[x\in S]\). My extended notation uses instead \([S]\) for the characteristic function, leading to the mysterious looking identity \([S](x)=[x\in S]\).

An integral sign with a horizontal bar through the middle indicates a mean value, meaning the ordinary integral divided by the measure of the region of integration (length, area, volume … in general, the result of integrating the constant 1 over the region). For example: